Typefaces based on tessellating patterns

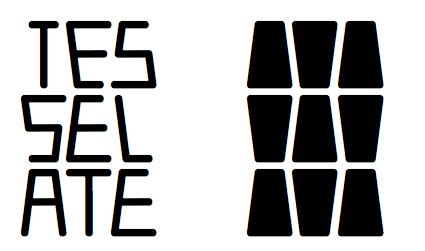
 It has been more than nine months since I posted here. During that time I have been designing typefaces, investigating what is possible using the OpenType feature Contextual Alternatives (calt). There are few typefaces that use this feature to alternate two character sets, perhaps because the results appeal to a niche market and serious type designers have better uses of their time than to design for a minuscule market. I like the feature because it gives interesting results when there is a geometrical connection between the two character sets that are alternated. My first use of the feature was to alternate letter sets that were based on trapezoids.

Notice that the trapezoids that serve as templates on which the letters are formed will tessellate. Tessellations were not on my mind when I designed this face. I was focused only on how the letters fit together in a word. However. I realized later that the template shape was part of a tessellation pattern, in the IH58 type. It can be constructed in several ways. The tops and bottoms can rotate 180º or flip to form neighbors. The sides can be C edges that rotate around their centers to form neighbors, or they can be considered glide edges. In terms of symbols, it could be CCCC, CGCG, CFCF, or GFGF. 

The typeface above did not exhaust the possibilities of trapezoidal letters, so I did a second family with a similar template but with letters that look very different.

Another trapezoidal shape that tessellates is formed by bisecting a regular hexagon. In this case I put the straight sides between letters and the interlocking part of the pattern on the top and bottom.

The trapezoid can be asymmetrical, as in the example below where the trapezoid was formed by cutting a rectangle into two equal parts with a slanted line. The pattern here can be formed by flipping the shape over the top and bottom and rotating it around the center of its sides. It fits the Grünbaum-Shepard IH49 classification. 

I followed up with letters formed with templates of rectangles and parallelograms that give a wave to a line of text. I did not think of tessellations when designing these, but the first template shape tessellates as a TCTC pattern (and also TGTG, CGCG, and G1G2G1G2) and belongs to the IH-66 class. In the second pattern, the tops and bottoms can be either center-point-rotation or translated edges and the sides can be either glided or flipped edges.

Using alternating characters one can design text that has a wave both horizontally and vertically. The template of the first of these is a IH-73 pattern (C4C4C4C4 at all corners, though it can also be formed as G1G1G2G2) and the second as an IH-71 pattern (fits both C4C4C4C4 and G1G2G1G2). The template shape in the second sample will tessellate in one orientation, two orientations, or four orientations. Only the four-orientation pattern is shown below.

Both of these typefaces are based on a template of distorted squares. 

I suspect a reason that I am willing to pursue this line of inquiry is because I like tessellations more than other type designers. No one else that I know of has designed multiple fonts from shapes that can form tessellation patterns.

For more information about these and other typefaces with alternating characters, see my type blog at nohyptype.blogspot.com

Grandpa's Amazing Tale: A Book of Mazes

It has been almost three years since I released Mazes Escher Would Like and after that book I did not expect to do another. There are so many maze books available that it is difficult to be seen. Also, the software I use is old and no longer runs on currently-available computers. And yet for some reason that I do not fully understand, in April of 2021 I decided to do one more.

As I was sorthing through old documents on my computer, I stumbled on an old story I had written.  I thought was funny and decided to illustrate it with mazes. Most of the mazes I used were old designs, but I did develop over twenty new maze designs for this book. 

Yes, I tessellated toilets and made a maze from the pattern. Below is another tessellation pattern I used.

The above maze would be trivial if displayed in a friendly typeface but it is one of the most difficult mazes in the book because it is so hard to follow the little passages that connect. It also posed a challenge for the way I construct mazes because the basic pattern is a grid of diamonds, so everything connects diagonally. 

The shape used above was also a challenge for my maze construction set. The basic shape is triangular, with one orientation adjacent to two above and the other with two below. I treated it as a maze of triangles and used two typefaces to render it.

Not everything is tessellation based. I used foot prints to form the walls of a maze that allows passages up and down, right and left, and diagonally.

The book is Grandpa's Amazing Tale: A Book of Mazes. It uses mazes to illustrate a silly story told by a grandparent to a granddaughter and involves time travel and dinosaurs, so it is both a story book and a puzzle book. It is intended for children in early elementary school.

It is published through Amazons's Kindle Direct Publishing and is available in paperback only at Amazon.

TessieSomeMore

In August I took a break for updating and creating alphabetic fonts to play with Tesselmanic! in an effort to create another tessellation font. With a lot of trial and error (mostly error), I was able to come up with over 40 designs that would fit into a typeface. I could not think of a clever name so settled for TessieSomeMore, indicating that it was a continuation of the Tessie series of fonts.

As I played with the various templates in Tesselmaniac!, I found that sometimes I would create something quite similar to what I had done in the past. One example is shape that resembles a butterfly. Below is the most recent version.

Looking through past efforts, I found something quite similar in TessieBugs. I kept the new version because it is so easily recognizable as an insect.

I noticed that if I added a straight segment where the wings touch, I could convert a tessellation that is IH68 to one that has six adjacent tiles and is type IH14.

Another insect design that resembles a past effort is this mite.

However, the old mite had six legs, so the new one is substantially different.

There are certain shapes that many people will stumble on if they play with tessellations enough.

Creating Escher-like pattern with the isohedral classification IH74 is challenging because there are not a lot of real-world objects that mirror both horizontally and vertically. Below is a stylized moth that fits the classification.

Again, the addition of a straight line where the wings touch turns it into a hexagonal figure. It retains all of its symmetry and is now IH17, another classification which is very difficult to use for Escher-like tessellations.

It is always exciting to find a new tessellating shape that is recognizable as an object. TessieSomeMore includes a shape recognizable as a toilet.

Another shape unlike any that I had previous found was one that resembles a creepy insect.

I tried to find interesting Escher-like tessellations in which the tile had two-fold rotational symmetry without mirror symmetry and came up empty. There are many real-world objects with reflective symmetry but very few with only rotational symmetry.

TessisSomeMore is available from myfonts. On the page linked, there is a link to a file that includes samples of all the patterns included in the typeface.

(See also this post at nohypetype.blogspot.com.)

TessieLetters and an unusual alphabet

I have finally returned to and finished a series of TessieLetter typefaces that complement the eleven Tessie typefaces (here, here, here, and here) that allow one to create tessellation patterns of birds, animals, bugs, and variety of other shapes. TessieLetters is made up of seven different fonts (each in solid and outline styles) that contain all the letters of the alphabet as well as the numbers. You can find them at fontspring.com: here, here, here, here, here, here, and here and at myfonts here.

One of the seven in the series contains only shapes that can be tessellated using a single key. Somewhat surprisingly, I was able to find ways to do the complete alphabet in this way, though some of the letters require a bit of imagination. (U, T, and G are not ideal.) A breakthrough came when I figured out a way to form the letter P. The same shape works for lower-case b, d, q and letters 6 and 9. Below is a picture of the entire one-key tessellating alphabet.


All these patterns would fit as Heesch types TTTT or TTTTTT. Many have symmetry that would allow them to fit in other Heesch types as well. Also, for several letters (such as f, h, m, n, s, and z) there are multiple shapes that work.

I am unaware of anyone else who has constructed an alphabet with this property.

In the spirit of the season:


(Cross posted at nohypetype.blogspot.com)

Tessellating Typefaces (part 4)

The addition of two more fonts of Escher-like tessellations at myfonts.com brings the total of the new tessie series to eleven. Four are of birds, one of other animals, one of bugs,  one of puzzle pieces, and four of everything else.

One of the recent additions is TessieBugs. It contains tessellating butterflies, moths, ants, and other creepy, crawly insects.

The other is TessieOddsNends, a hodgepodge of things that did not make it into any of the other ten faces.

Each contains two styles, a solid style that must be colored in order to see the shapes (after all, a tessellation fills the plane with no gaps or overlaps), and an outline style that can be used alone or layered over the solid style.

Tessellating Typefaces (part 3)

Three more tessellating typefaces have been added to Myfonts.com. Included is a fourth typeface of birds, TessieXtraBirds. When playing with TesselManiac and before it TesselMania, I have had the tendency to see bird possibilities much more readily than other shapes.

The other two typefaces have a variety of shapes and no clear theme as their names indicate. Below is a poster for TessieMoreStuff. The plane shape has four edges that are all shaped with identical center-point rotation. The poster has them in three different tiling arrangements.

The musical notes from TessieMiscellaneous are a based on a triangle. It is a pattern unlike any that I have seen elsewhere.

Each family contains two styles, a solid style that must be colored and an outline style that can be used alone or layered over the solid style (as shown in the two top illustrations). Each has a sample file that shows what key combination are needed to produce the various patterns that are possible.

(Cross posted at nohypetype.blogspot.com)

Tessellating Typefaces (part 2)

Three more tessellating typefaces have been added to Myfonts.com. Included is another typeface of birds, TessieMoreBirds. Many of the bird shapes in this typeface are of swimming birds. I noticed that combining two of the swimming birds in the tiling below would result in both swimming and flying birds (shown on the right).  (The flying birds will not tessellate alone.)

 TessieSpinners has a variety of shapes that have suggest spinning or rotating.

Finally, TessieAnimals has tessellating shapes that resemble fish and mammals.

Part 1, with three other tessellating typefaces, is here.

(Cross posted to NoHypeType.blogspot.com)